Solar Empire

Every so often I’ll see an article extolling the future of driverless cars and threatening the obsolescence of people owning and controlling their own vehicles. The fact that all of these articles are playing on fear is telling: They aren’t enticing customers, they are threatening them. Nevermind the technical issues surrounding attempts at autonomously navigating a chaotic and complex world: My problem with the way “driverless cars” are being pushed is that they’re being *pushed*. They aren’t being sold to customers the way any of industry’s great success stories are being sold. Think about the personal computer. Think about the internal combustion engine.

Think about the way Windows 7 was sold to customers as a personal operating system, vs. the way Windows 10 was pushed on them (breaking computers and wrecking data in a process that I’m amazed Microsoft got away with legally). The way users are constantly being threatened with “dumb info-appliance terminals that you can rent as a service”, aka “you don’t need that much computer.”

—–

With any successful product which actually changes the world, people have an incentive to buy it because it is a tool they can use to solve their problem. It is *their* tool which *they* can use to solve *their* problems. If someone comes up with a driverless system that lives in the dashboard of a car the user owns, (and therefore *controls*) that the user can turn on and off at will – a sort of advanced cruise control (and given the limitations of computers understanding the world, that will be *necessary*): That would be a way to sell automated cars to customers. Pushing for a utopian (almost all utopias are dystopias because they are fundamentally disempowering) future where “you don’t need a car, you can rent from some fuedal overlord who owns all the cars” is exactly as attractive to me as the people pushing dumb terminals “you don’t need a whole personal computer – what are you going to do with it? Just rent applications from the cloud.”

In one presentation by a grad student working on an avionics problem, a business jet crash was outlined: A pilot was trying to land a business jet but couldn’t deploy the landing gear. The jet continued circling the tower while the pilot fought with his computer. Apparently the avionics were programmed so that the pilot couldn’t deploy the landing gear if the flight computer thought it was above a certain altitude. The alitmeter wasn’t working because the pitot probe got clogged with something while flying. Eventually the pilot ran out of gas and had to make the attempt: He belly slid across the runway and spun out into a hangar.

The lesson the person doing the presentation *wanted* us to take from this was “we obviously need *smarter* avionics that can combine two or more pieces of info!” A professor from the combustion lab stood up and asked the obvious: “Why didn’t the pilot have an override?!” The grad student stared at him like he had grown two heads: “Why would you even *want* that?!”

There seem to be two deep philosophies at war in how products and systems are being designed in the modern world. In one, technology is supposed to empower the user to do something, in the other technology is supposed to control the user and funnel him into some pattern of working or living that the designer wants. One is something people will actually buy of their own free will. The other is something they’ll have to be forced into somehow. (Or perhaps, one is something the operator would buy, the other is something someone who thinks they ‘own’ the operators might buy if they are sufficiently arrogant about “replacing them”.)

There seems to be a fundamental lack of respect for the customer, owner, operator, pilot, what have you.

Take a look at this! This is all kinds of awesome.

One thing bugs me about these multi-rotor vehicles though, and it’s been bugging me the whole time various inventors have been showing off their stuff: The designers are always running things off of batteries! That vehicle couldn’t have had much more range than what was depicted on batteries.

That’s fine for an awesome demo that flies around the block. But there is absolutely nothing, in terms of engineering or technology, preventing these from being practical vehicles: Except the designers’ strange refusal to put a combustion-driven generator (microturbine, lawnmower engine, whatever!) on it. With it, you have payload capacity, endurance, and range. Without it, it’ll just be the *suggestion* of a flying car.

(I realize that if you took any of these in the direction of a practical vehicle, the full fury of $bureacracy would descend upon the hapless inventor: But that’s the real problem, isn’t it?)

*This* is something that I wish I had found affirmation for before (though I suppose I’ve been stubborn enough on that front not to have needed it). I’ve always sort of believed this, as one of my ‘personal heresies’ – things that you know are true that society doesn’t let you acknowledge. If you really want to understand something – to know fundamentally how it works, not just to stack someone else’s black box into the assembly of whatever you are working on, you pretty much *have* to reinvent the wheel. If you reinvent the wheel, it’s *your* wheel. In my case, I can reconstitute how something works and remember it far more effectively if I’ve done that at some point in the past, than if it were just some random magic-trick I couldn’t take the time to understand.

From Longuski, James, Advice to Rocket Scientists

Recently I’ve purchased a wonderful device: An Nvidia Tesla M2070 GPU.

Over the past 8-10 years, a new field in high-power computing has been developing: that of using the computational power of graphics processing units to perform numerical computation.

The requirements for rendering detailed and complex images in real-time led to the development of special purpose processors for graphics cards. Specific applications then drove the development of GPUs intended for general purpose computing. These processors, while limited in some respects relative to supercomputing clusters with large numbers of independent cores, can nevertheless perform floating point operations at a comparable pace.

The NVIDIA tesla M2070 is rated at 515 Gflops (billion floating point operations per second), and has 6 GB of memory. I bought mine from e-bay for $100. The bitcoin bubble (for which a lot of these GPUs were repurposed) has burst, and a lot of powerful hardware is being resold right now for peanuts!

Eventually I want to be able to do this: https://www.youtube.com/watch?v=vYA0f6R5KAI

That wasn’t done on a supercomputer. That was done on a single GPU. That is how much power that is within easy reach of anyone operating an (appropriately powered, appropriately cooled) desktop PC these days.

So far, I’ve been following the tutorials for writing SIMT/SIMD programs using the CUDA API. Another API that is used to program these GPUs is OpenCL, which I’ll get to after I get through the CUDA tutorials.

So far, the tesla, along with my graphics card that does actual graphics, have been able to accept command kernels, allocate, deallocate, and transfer memory. It performed 4.1943E16 integer operations in about ten seconds – it took far longer just to randomize the integer vectors on the host side than it did to run the operations on the device side. (edit: on reexamination, it couldn’t possibly be running this calculation to completion. I think I’m overwriting the execution in a host loop – will have to check…)

One problem that I will have to address though is the heat generated by this GPU. Even idling, the card gets rather worrisomely hot. When I start stress-testing it, it begins to act weird about a second or two into the computation and gets hot as an oven. (Solder starts to melt at 230-ish C, so I’d better watch out!) Conservation of energy says that I’m spending electricity as if I have an always on toaster. There are heat-sink ribs on the bottom of the device that are oriented such that it looks like they expect a fan to blow air across the length of the card. I’ll have to figure out how to design a bracket that will hold a fan to the back of the case so that I don’t burn the card up in future use.

Anyway, with luck (lack of migraines), and frantic typing, I should have a CFD engine for structured grids banged together. Then I want to investigate this octree-based grid that SpaceX is using to such amazing effect in their modeling efforts.

I’ve got to publish updates occasionally, just to keep the blog dream alive.

Recently, I’ve been learning OpenGL. There are some nice OpenGL implementations in python, making the development and testing of algorithms pretty easy. I need to port all this stuff over to C++ (sort of halfway started into that), so that I can start writing more serious applications.

Pyglet is a python windowing library that lets you use OpenGL to draw to a screen.(The amount of stuff you have to go through on modern PCs/programming environments to start drawing stuff to a screen is immense. Once you have ‘drawing to a screen’ down though, you can start getting that rich visual/dynamic feedback that makes programming (more so) an intrinsically rewarding activity.)

Disclaimer: This is my personal blog. Any opinions presented here are personal opinions, not affiliated with my professional work. All work shown here was done on my own time with personal resources.

Elon Musk made a speech at the International Astronautical Congress outlining SpaceX’s goal for the sort of vehicle they want to build as part of their Mars plan.

It’s here on Youtube. https://www.youtube.com/watch?v=IFA6DLT1jBA

I would recommend watching it: It’s an interesting presentation. Only partially flashy imagery, it contains some technical details about their mission idea and what they are working on to build up to it.

The vehicle described is an extremely large, extremely lightweight (for the size and propellant mass) vessel. It has to be to have a chance of doing what it is proposed to do (fly from LEO to Mars with only a single chemically-propelled stage). (The goal is for the booster to have only 4% inert mass!) Needless to say, there will be a great many engineering challenges to build the vehicle described in the presentation. It will be interesting to see exactly how far composite construction can be pushed, and if these mass-fractions can be attained, much less result in a vehicle that can withstand aerobraking (not just at Mars, but returning to Earth). It will be interesting to see if the problem of pushing a very large vehicle with a great many rocket engines can be solved. (You get noise problems either way: With many small engines, or with a few very large engines.)

But how does it hold up on the basic physics? One of the things that caught my eye during the presentation were the extremely short travel times to Mars. Chemically propelled spacecraft and probes usually launch from Earth at specific times, coast to Mars, and enter Mars at specific arrival times to minimize the delta-V, which governs the propellant cost of the mission. Mass margins on chemically propelled rockets are tighter than a swiss watch, and no one launches outside of certain very narrow minimum delta-V launch windows. These windows, for both type I and type II trajectories tend to result in times of flight much longer than 80 to 100 days: Usually 8.5 months is given as a ballpark. Somewhere between 200 days and a full year.

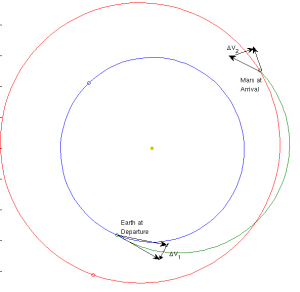
This is an example of a transfer orbit. It isn’t a good one, but it is plotted for example’s sake to show where you maneuver and how to get from Earth to Mars.

This is an example of a “porkchop plot”, which shows totally propulsive delta-V’s for Earth-Mars transfers. It shows the delta-V as a function of departure and arrival times.

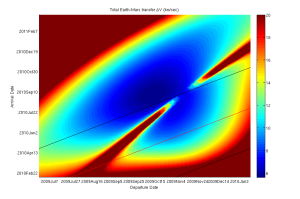
Porkchop plot for Earth to Mars, total Delta-V, 2009 departures. The three lines are 100, 200, and 300 day travel times respectively.

This bothered me, because SpaceX is a rocket company. They can’t not understand orbital mechanics and do what they do. It would be highly incongruous to design a rocket, and mess up the orbital mechanics – which is where the design of space missions tend to start! So, odds are they aren’t pulling numbers out of nowhere. But what assumptions are they making that let them do that?

I think I’ve figured out some of what you would have to assume to actually get those transit times.

First: The upper-stage spaceship: The desired inert mass, propellant mass, and thruster Isp are given. These determine how much delta-V the spacecract can develop before it runs out of fuel. Tsilkovsky’s rocket equation gives the following.

(m_final/m_total) = (1-m_prop/m_total) = exp(- delta-V/v_e)

With 450 tons of cargo, the vehicle only has 5.4 km/sec of delta-V. The spacecraft has to make several maneuvers to reach Mars.

1. It has to escape from Earth from the starting orbit.

2. It has to thrust to transfer from a near-Earth solar orbit to a Mars-Transfer orbit.

3. It has to somehow enter a Mars orbit from the transfer orbit (more on this later).

4. It has to land on the surface propulsively.

To escape Earth from LEO, you need 3.25 km/sec of delta-V. This only leaves 2.1 km/sec left over. This isn’t enough to make the transfer to Mars from even the most favorable conditions on even the slowest of trajectories.

Suppose you don’t carry the full load of cargo though: If the vehcile was only carrying 100 tons, then it has 8.1 km/sec, which leaves 4.8 km/sec of delta-V left over after escape. This could enable the vehicle to make some of the easier transits and still have a tiny bit of delta-V left over for landing.

How does the vehicle get off the transfer orbit, into Mars orbit, and land? This mission concept must assume that all the delta-V on Mars entry is taken care of by aerobraking. The vehicle will be significantly lighter when it reaches mars, and so might have some advantages there: Large surface areas and low mass gives a ballistic coefficient that <i>might</i> allow it to shed both the transfer orbit velocity (dV2) and the velocity it will pick up by falling into Mars’s gravity well (about 4.9 km/sec). The delta-V’s for aerobraking for the short travel times can be quite high though: 5 + anywhrere from 5-10 km/sec for these faster orbits. I’ll have to think about how the thinner atmosphere will change things. (For reference, the EDL stage of the Curiosity lander hit the Martian atmosphere at only ~5 km/sec. It must have matched the solar-orbit speeds with Mars very well at the end of it’s transfer, which you can only do with low-energy transfer orbits.)

Propulsive landing: You want some delta-V to make the landing. 1000 m/sec is a pretty tight margin, especially since Mars gravity is higher than the moon’s. You don’t have to deal with propulsively canceling orbital speeds, but you do have to apply positive control to land and stop falling.

But how would you do it in 80 days? Suppose instead of escaping Earth from LEO, you launch into a near-escape elliptical orbit. Tankers would have to follow the vehicle around on the elliptical orbit and fill it there. When the vehicle reaches perigee, it can make a small delta-V to escape Earth, and have most of the delta-V left over for Mars transfer and landing.

Below are some plots: They are plots of the “launch windows” between Earth and Mars, in terms of delta-Vs. They are the porkchop plots from before, but skewed 45 degrees to be put in terms of transit time. The first is the Earth to Mars tranfer delta-V. This is the only one the spaceship would be making. The second is the Mars-Transfer to Mars solar orbit delta-V. This, plus ~5 km/sec is what you need to shed on aerobraking. If you were trying to enter a controlled orbit about Mars, you would need to spend the total delta-V (third plot), and maneuver in Mars orbit (with some ability to finesse due to gravity capture, etc).

Color indicates delta-V in km/sec

So ~100-120ish days isn’t outside the realm of physical possibility after all, if you can aerobrake away your Mars entry delta-V, and if you take less cargo, and if you launch from a high Earth orbit instead of a low Earth orbit… Okay, so it is a little crazy: It has absolutely no margin for error. But it’s not impossible.

(PS 450 tons, 100 tons – these are very large masses relative to anything we fly today, but what matters in interplanetary flight is the mass relative to the propellant (landing is another matter). The vehicle is stated to have 150 tons of inert mass, 1950 tons of propellant, and up to 450 tons of cargo. The Isp of the engines is 387 sec, giving 3743 m/sec of jet exhaust velocity.)

For reference:

Earth escape starting from LEO: 3.4 km/sec

Earth escape starting from near Earth but not orbit: 11 km/sec (also what you pick up when you return to Earth and fall into it’s gravity well. A very heavy re-entry loading seen during Apollo.)

Mars entry (or escape) from the surface: 5 km/sec

Mars entry (or escape) from low Mars orbit: 1.45 km/sec

Typical total Earth to Mars transfer delta-V given that it is minimized (leads to 200+ day travel time): 5.5-8 km/sec.

For playing with the travel times and launch dates, see the plots above:

Also, these plots assume you are only doing a two-impulse maneuver. Trajectories with fly-bys, or trajectories with 3 or more impulses, or low-thrust trajectories require solving optimization problems.

So, I am now an official rocket scientist. A great many things have happened over the past month.

1. I graduated from Georgia Institute of Technology with a PhD in aerospace engineering. My thesis was on certain features of channel wall erosion in Hall Effect Thrusters.

This is an image of a Hall Effect Thruster: A type of highly propellant-efficient electrostatic thruster for spacecraft.

2. I moved to Cleveland OH from Atlanta GA. I survived. This time all of my furniture survived the trip.

3. I am now renting an awesome house in Parma Heights.

4. I have started work as an “Aerospace Technologist” at NASA Glenn Research center. I will be working on the European Service Module portion of the Orion project.

Below is a photo of my swearing-in ceremony.

I now have a lot of time in the evenings though, and on weekends. I’ll have to get into the habit of seriously pursuing my hobbies again.

If you’ve ever seen one of those old 60-70s era space-exploration paintings, you might have been looking at the artwork of Chesley Bonestell. I’m pretty sure a few of those paintings invaded my brain back in my childhood, though I didn’t know the artist at the time.

Check out his stuff:

https://www.google.com/search?q=chesley+bonestell&hl=en&biw=748&bih=753&site=webhp&source=lnms&tbm=isch&sa=X&sqi=2&ved=0ahUKEwj_u4aJ8KLNAhXJFT4KHQlnDvoQ_AUIBigB&dpr=1

This guy (Dave Robinson) also has a lot of very nice spacecraft/space-exploration artwork:

http://www.bambam131.com/

http://www.eso.org/public/news/eso1615/

Three new planets, similar in size to Earth, and orbiting close around a red-dwarf star have been found by the European Southern Observatory in Chile!

One of the trends coming out of the extrasolar planet searches of late is that there are actually a lot of planets orbiting in the green-bands of red-dwarf stars. It used to be thought that because the dwarf stars were cooler and had closer and smaller habitable bands, that there was very little chance of an Earthlike planet forming around these stars. It turns out that the red-dwarf stars seem to have much closer packed systems though, so it all evens out.

Red dwarf stars (class M) make up 80% of the stars out there, so this is very good news. https://en.wikipedia.org/wiki/Stellar_classification

I don’t have exact numbers, but for a WAG the other day, based on stellar densities, http://abyss.uoregon.edu/~js/ast122/lectures/lec26.html, of about 1 star per cubic parsec within the solar neighborhood, there should be close to a 100,000 stars within 100 LY of the solar system. (Volumes grow fast – note to sci-fi writers.)

Edit: Wikipedia gives 0.14 stars/pc^3 in the solar neighborhood, which would lead to 20,000 stars in a 100 LY radius sphere. This accords better with the closest stars (26 in a 12 LY sphere). https://en.wikipedia.org/wiki/Stellar_density. Nevertheless, space is big, and as we are discovering, it is far from empty!

My server is dead, it seems. The hard drive won’t boot. I had warning of this problem and backed up all the essential files to the NAS prior to the drive failure sometime last week.

I suppose I’ll be shopping for a drive tomorrow. I depend on the server to coordinate all my Zotero Papers between my myriad computers. Won’t spend too much time on it, but hopefully will be able to restore everything by the weekend.